Section 2.3 is entitled “Models of Motion.”
This section has quite a bit of words about history and
physics and such. If you’re here for the numbers, you’re going to have quite a
bit of exposition to get through. I’d apologize, but history is cool and
physics is cool and together they are awesome.
The study of motion has been a thing for thousands of years.
Humans are fascinated particularly by the motion of planets, and have tried to
model their motion for years (as far back as the Babylonians, who made the
first recorded observations regarding planetary motion).
Greek astronomers Hipparchus and Ptolemy believed the earth
was the center of the universe and that everything (including but not limited
to the sun, the moon, and other planets) revolved at constant velocities in circular
paths around the earth. Then again, pretty much everyone believed the earth was
the center of the universe. Well, everyone except Aristarchus, it seems.
 |
| My interpretation of their confrontation. These would be their exact quote, of course. |
As math got better, Hipparchus and Ptolemy realized the
circular paths part of their theory wasn’t true. To account for this, they
developed epicycles, which are smaller circles around the larger circle. The
center of the smaller circle rotated in a circular path, while the other
planets rotated in the smaller circle. So the planets would move around the
epicycles as the epicycles moved around the earth.
 |
| E means earth. P means planet. C means center of smaller circle. |
The next major improvement came in the 16th century, when
Copernicus stated the earth was not the center and rather the sun was the
center. This was a major change in the way people thought in that time, but it
made the epicycle calculations much easier to do so I guess it all worked out in
the end.
In 1609, Tycho Brahe (the most fun physicist name to say, if
I do say so myself) made observations about the motion of planets and Johannes
Kepler created the three laws of planetary motion:
“1. Each planet moves in an ellipse with the sun at one
focus.
2. The line between the sun and a planet sweeps out equal
areas in equal times.
3. The squares of the periods of revolution of the planets
are proportional to the cubes of the semi-major axis of their elliptic orbits”
(38).
 |
| Coincidentally, Tycho Brahe also had one of the more impressive moustaches (Wikipedia) |
The great thing about Kepler’s laws was that there was no
more need for epicycles. Also, the idea that planets and moons and such
interstellar bodies were traveling at a constant speed were thrown out as well.
Our next stop on the history of astronomy train is Newton,
who made three major advances when it came to planetary motion. First, he
created calculus, which helped to derive and thus validate Kepler’s laws.
Second, he created the laws of mechanics. In particular, we’re talking about
his second law (force is equal to mass multiplied by acceleration), because
then the study of motion could be simplified down to differential equations.
Third, he postulated the universal law of gravity, which mathematically
described gravity.
In 1919, Einstein proposed the theory of relativity, which
explained gravity as the result of the curvature of four-dimensional space-time.
 |
| Gravity makes sense now...I think. (NASA) |
In the 20th century, physicists have realized there are four
fundamental forces of the universe: gravity, the strong nuclear force, the weak
nuclear force, and the electromagnetic force. However, physicists strongly
believed all of these forces are unified. Quantum mechanics links three of the
forces (minus gravity), and there is a disconnect between the way relativity
explains the universe and how quantum explains the universe. I could create an
entirely different post about the differences and the way each explains the
universe, but this is math and I shall continue on.
Recently (but not recently, since we have the M-theory now),
some physicists have created the string theory as a way to unify relativity and
quantum mechanics. The shorthand version of string theory says that particles
in the universe act as strings that move in 10-dimensional space time. (M-theory
postulates 11 dimensions, but we’re not going to overturn that rock.)
 |
| Because 4 dimensional space-time is too mainstream. (particlecentral.com) |
However, at this point string theory (superstring theory,
M-theory, what have you) cannot be validated. Physicists are working on an
experiment to test this theory, but for now we will just have to wait.
*
The previous history lesson presented about six mathematical models or theories
of planetary motion (I counted, but I don’t want to recount and list them for
you, so just take my word for it). With each updated model, there was a more
general application for the motion of planets. This is an elaborate example of
what should happen whenever a mathematical model is created or used – when we
become better educated, we should change the theories to make them better.
Looking specifically at Newton’s theory of motion, we’re
going to dive into numbers now. At this point, the book is limiting us to one
dimensional motion. Hey, at least they didn’t just throw us in the metaphorical
pool of ten-dimensional space time math problems and say, “Swim or die.”
 |
| I don't know about you, but I'd probably drown. |
We’re going to look at a very general example of a ball
bouncing straight up and down in the air.
 |
| You can tell this is going to be exciting because the background is yellow. |
Let x be the distance between the ball and Earth’s surface.
Let m be the mass of the ball. From first semester calculus/physics, we know
that the velocity is the first derivative of the distance and acceleration is
the second derivative of distance (and thus the first derivative of velocity).
Newton’s second law says the force on the ball is equal to its mass times the
acceleration it is falling at. Since the ball is under the influence of
gravity, the equation is as such:
where g is the gravitational constant.
Knowing all these things, Newton’s second law on the ball becomes:
Since this is a differential equation,
this can be solved. I’m not going to solve it step-by-step but instead show you
the answer:
The initial velocity is v_0 and the
initial height is x_0.
With this example, we took on the
assumption that gravity was the only force acting on the ball. In real life,
this isn’t true. There are quite a few forces acting on a ball that is falling
to earth. We’re going to talk about air resistance now.
Here are three facts about air resistance:
1. If the velocity is zero, there is no
motion and that means there is no resistance.
2. Resistance will always act opposite to
the direction of an object’s motion. This means resistance will have a sign opposite
of velocity.
3. Resistance is very complicated and no
one law can describe all cases. There are many cases (because this is reality,
where stuff changes every day).
 |
| You can quote me. I'm deep. |
For now, we’re just going to focus on two
different cases. For these two cases, we’re going to solve a differential
equation, look at terminal velocity (more explanation in a second), and solve
for the displacement. There’s quite a bit of math involved (to balance out the
words at the beginning, I suppose).
Case 1: r is a positive constant
Forces can be superimposed, so our
equation starts off as the following:
This equation is separable and thus
solvable. Skipping over those steps (do it yourself for the practice, I suppose),
the solution is
C is just a constant. Now, if we look at
the limit as time goes to infinity, we are left with –mg/r, which is what we
call terminal velocity. This basically means that if a colored pencil was
dropped from the top of a building, at some point the pencil will stop
accelerating and will fall at a near-constant velocity based on its mass and
air resistance.
Finally, let’s solve for displacement:
A is another constant due to the
integration required to find displacement.
Case 2: magnitude of the resistance is
proportional to the square of the velocity
In the language of math, our second case
is as follows:
With Newton’s law, this becomes
The book uses tricky calculus to solve
this by substituting v and t for other things. If you believe the book (and if
you believe me), then I’m going to skip these steps.
The solution is
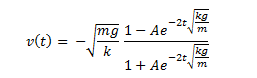 |
| Do you vaguely understand the situation, now? |
As time goes to infinity, the terminal
velocity is modeled as
Because of the monster of a solution
above, integrating to find displacement would just be crazy.
(Caption: They know what they’re talking
about.)
Instead, we’re going to use the chain
rule.
That is all for this section, ladies and
gentlemen! I’m trying to have fun with the blog because for the most part, math
summaries can be pretty dull. I hope you’re enjoying it as much as I am.
For more information about air
resistance, visit this page. It looks legit: http://physics.info/drag/
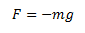





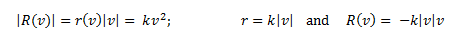



No comments:
Post a Comment